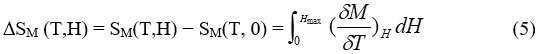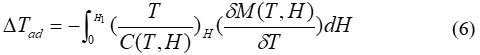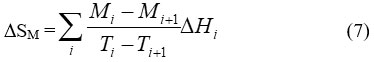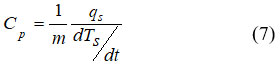Introduction
One of the human goals is getting an environmentally friendly technology in order to overcome the pollution probelems. As it is well known, the capacity use of the gas refrigerators (Freon refrigerators) is one of the main reason for the harmful changes in climate by increasing the Ozone hall. The potential success in synthesis a new materials and studying theie magnetic properties ebable us to use them in the cooling system. Magnetic refrigeration is one of the effective cooling technology principally depends on the magneto-caloric properties of these new materials to reach extremely low temperatures comaprable with the conventional refrigerators relying on the selected design of the system.1 These new materials are clean and environmentally friendly technology in order to reduce the harmful changing in the climate. From technological point of view, magnetic refrigeration is clean technology that made scientific revolution in cooling systems and on it need to good commercialization. Magnetic refrigeration was the first method developed to attain the temperature below about 0.3K (this very low temperature was found in 3He refrigeration with 3He vapors).1
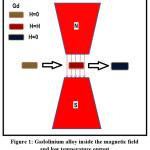
Figure 1: Gadolinium alloy inside the magnetic field and low temperature output
As a result of application of sufficiently high magnetic field on a magnetic material, the reorientation of thier magnetic moments are achived. Adiabatic application of magnetic field may lead to the increase of temperature and with removing this field the temperature decreased. The process of warming and cooling resulted from the application of an external magnetic field or removal it is termed as magneto-caloric effect ‘MCE’. The basic idea of magnetic refrigeration is shown in fig. 1. When we apply a magnetic field on magneto-caloric material, for example Gadolinium alloy,1-3 thermodynamical process is occurred and resulting in the drastic decrease in temperature as we will see in details.
The magnetocaloric effect can be mathematically discussed as the following. At constant pressure, the magnetic entropy of a ferromagnet (FM) relies on both magnetic field ‘H’ and temperature’T’, and the value of entropy is the sum of the lattice (Slat) and electronic (Sel) entropies and the magnetic entropy(Sm),
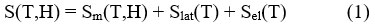
Two relevant processes are explained to define the thermodynamics of the MCE:
– According to adiabatic application of magnetic field (i.e., the total entropy does change) in a reversible process, the magnetic decrease in the entropy obtained, but the total entropy remains constat,

This adiabatically increase in temperature can be conceptualize as the isentropic difference between the S(T,H) functions giving the value of the MCE for the underinvestigation material,
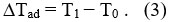
– According to the isothermal application of magnetic field (Temperature is fixed), the decrease in the total entropy achieved as a result to the decrease in the magnetic field, and thus the change in entropy process is termed as the following
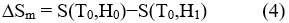
Change in the adiabatic temperature “∆Tad ” and change in the isothermal magnetic entropy “∆Sm ” are peculiar values of the MCE. They are functions of the following parameters’ the initial temperature,T0, and the magnetic field variation ∆H = H1−H0. The increase of the field may lead to the increase of the magnetic order and thus occurring , decrease in the the magnetic entropy, and ∆Tad (T,∆H) becomes positive (rising in temperature) with negative ∆Sm(T,∆H). In the reverse process, as a result of reducing field, a decrease in the magnetic order achieved and negative ∆Tad(T,−∆H) appeared with positive ∆Sm(T,−∆H), causing the cooling of the under investigation material.
The magnetic entropy∆Sm(T,∆H) and ∆Tad(T,∆H) are mathematical description of MCE process as shown by the following equations (5) and (6)4-5
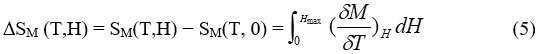
It is quite clear that the change in the magnetic entropy depends on the derivative of magnetization with respect to temperature at certain magnetic field and on the variation in the magnetic field. According to the following thermodynamic relation,5 we obtain an expression that characterises the magnetocaloric effect, the infinitesimal adiabatic temperature change,
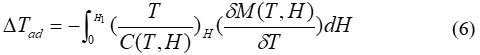
Valuable information about the behaviour of the MCE in materials can be obtained from analysis of Eqs. 5 and 6:
At constant magnetic field, a decrease in magnetization of paramagnets (PM) and simple ferromagnetic FM materials achieved with increasing temperature. In Ferromagnetic materials, maximum value for the derivative of magnetisation with respect to temperature is achieved at Tc and from the other side ∆Tad(T,∆H) shows a peak when ∆H tends to zero.6 DSM can be approximated by measuring magnetization as a function of temperature at certain magnetic field according to the following relation7:
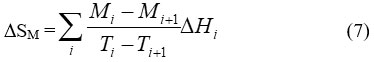
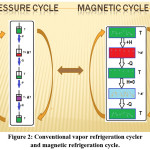
where Mi and Mi+1 are the magnetization values measured at temperature Ti and Ti+1, respectively, in the presense of certain magnetic field H. Based on the above mentioned equations, one can say that magneto-caloric effect can be promoted by1 applying a large magnetic field with a small heat capacity as well as with a large change in magnetization vs temperature, at a constant magnetic field . Thermodynamically, magnetic refrigeration cycle is similar to the Carnot refrigeration cycle, where the magnetic refrigerator cycle depends on the increases and the decreases in the magnetic field instead of the increases and the decreases in the pressure in which the Carnot refrigerator cycle depends.1 Comparison between magnetic refrigeration and conventional refrigeration cycle is very important to evaluate the magnetic refrigeration and understand how magnetic refrigerator work. As it is shown in schematic diagram in Fig.2, there is a similarity between conventional and magnetic refrigeration cycle.1
Figure 2: Conventional vapor refrigeration cycler and magnetic refrigeration cycle.
In adiabatic magnetization, a magneto-caloric material is placed in an insulated environment, then, as a result of increasing an external magnetic field (+H) the magnetic moments are aligned in the direction which leads to increasing in the material temperature and decreasing in the magnetic entropy and heat capacity of substance. The total entropy does not change because there is no loss in energy and so heating up is observed (T + ΔTad).
For the conventional vapor refrigerator CVR cycle the gas is compressed by applying pressure P and similarly there is no loss in energy and so heating up is observed (T + ΔTad). In isomagnetic enthalpic transfer: we can remove the generated heat somehow. Prohibiting the reabsorption of heat by the dipoles, we keep magnetic field constant and once cooling is enough attained we separate the magneto-caloric substance and the coolant. In adiabatic demagnetization: The total entropy remains constant as a result to return to another adiabatic (insulated) condition. However, in this stage the decrease in the field is obtained, the thermal energy leads to magnetic moments to overcome the field, and hence cooling occur, i.e., change in the adiabatic temperature. Energy transfers from thermal entropy to magnetic entropy (as shown in Fig.2, disorder of the magnetic dipoles is appeared). Based on the isomagnetic entropic transfer: We keep magnetic field fixed to prohibit the warming up again. Because of the difference in temperature according to our design, heat energy emigrate into the magnetocaloric material (+Q).
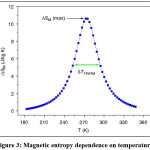
Figure 3: Magnetic entropy dependence on temperature8
How to evaluate the relative cooling power RCP is an important parametre and is an indication to the resulting magnetic cooling efficiency [8] of the magneto-caloric material and it can be simply considered as the magnitude of DSM or DTad multiplied by the full-width at half maximum (dTFWHM).3,9 DSM, DTad and dTFWHM are shown in Fig. 3 where the change in entropy is plotted as a function of temperature. The change in entropy could be deduced using equation 3 from magnetization – temperature dependent measurements. On the time being the conventional vapor compression refrigerators are used for cooling applications; such as refrigerators and air conditioners AC. However, the compressing / expanding processes efficiency is low in the conventional gases and costs for 25% of housing applications and 15% of commercial power consumption.8 In the conventional vapor compression refrigerators, the application of different gases for instance, chlorofluorocarbons (CFCs) and hydrochlorofluorocarbons (HCFCs) is dangerous because they damage our environment. Alternatively, searching a new cooling system is crucial task to keep our environment safe and clean so that the progess in magnetic cooling based on MCE is of the great interest.10 Now, the question is why MR is promising task for cooling technology. The answer is given in the Phan section in ref.8 as following;
First, important task is the efficiency of the cooling in such MR is higher than CVR where the attainable efficiency is in the range of 30-60% while the cooling efficiency in CVM according to a Carnot cycle is only 5-10%. Second advantage of the MR is the compactness where solid substances are used as working materials and thus they do not occupy big place. Third, the materials used in MR are not toxic or harmful where no global-warming gases are produced in the process of cooling and therefore they are considered as an environmentally friendly cooling systems. Our main goals in the present proposal are introducing advanced and cheap materials with enhanced properties for cooling systems and developing a new generation of refrigerators and cooling systems environmentally friend to keep our environmental clean and safe.
In this review we highlight the scientific efforts to find the best alternative materials to be used in magnetic refrigeration applications; for example, Gd-Si-Ge alloys11 Ni–Mn–Ga alloys,12 Mn–As–Sb alloys,13 La–Fe–Co–S alloys [14], Mn–Fe–P–As alloys,15 TmGa compound,16 Praseodymium alloyed with nickel (PrNi5),17La–Ca–Sr–Mn–O manganites (3d-4f oxides).18 This review is consists of three sections; I. Synthesis of MC materials, II. Crystal structure of MC materials and III. Characterization and applications of MC materials.
Synthesis of Mc Materials
The MC alloys were prepared using common Arc melting method. Stoichiomrtric starting materials is placed in water cooled crucible and the firing is held under the argon atmosphere. The purity of all the initial materials is in the range of 99.9%. The initial materials with the proper stiochiomertic ratio are sealed in quartz tube after filling it with high purity argon gas. The melting process is repeated several times with shaking the crucible in order to obtain good homogenous alloy.19 The CeSi ingot was synthesized by Wang et al.,20 using arc melting method with the proper stoichiometric precursors (Ce and Si) on a water-cooled copper crucible in the presence of argon gas atmosphere. The selected purity for the intial metals in this work are higher than 99.9. Milting the sample for several times is important to ensure homogeneity of CeSi. The annealing temperature was 1223 K for 7 weeks.20 Md Din et. al.,21 prepared the polycrystalline alloy NdMn1.7Cr0.3Si2 using arc melting in the presence of Argon gas followed by annealing for 1 week in an evacuated quartz tube at temperature of 900°C.
Polycrystalline DyCuSi alloy was prepared in ref.,22 by arc melting technique in the presence of Argon gas. On similar way, we can warp the obtained ingots by molybdenum foil to be sealed in evacuated quartz tube. The annealing temperature was 850°C for 1 week and with liquid nitrogen quenching temperature. The same method was used to prepare Er1-xTbx.Co2 alloys (x=0.8 and 1) using arc furnace melting in the presence of an argon gas, see ref.,23 The alloys were then fired at 800°C in high vacuum quartz tube for 1 week. 3d-4f oxides (rare earth–transition metal oxides) of perovskite like structure have magneto-caloric property and could be used for MR. There are different methods for synthesis of such oxides for example; solid state reaction, coprecipitation method, sol. gel. method, hydro-thermal method, microwave radiation method. According to work reported by Phan et al.,24 La0.65Sr0.35MnO3, La0.6Sr0.2Ca0.2MnO3, La0.6Sr0.2Ba0.2MnO3 and La0.7Ca0.06Ba0.24MnO3 compounds were prepared by the standard solid-state method from a proper stoichiometric pure oxides of La, Sr, Ca, Ba, and Mn and calcinated in furnace at 1250°C temperature for 16 h and followed by regrinding and pressing the powder in pellet form and finally sintering at 1350°C for 18 h.
Zhong25 used modified sol–gel method in preparation of the polycrystalline La0.67Ba0.33MnO3-d withperovskites-like structure. In their work, they dissolved proper amounts of La2O3, BaCO3, and Mn(NO3)2 in dilute HNO3 solution at 333 K. Then, they added the equivalent amount of citric acid with some ethylene glycol to generate a completely homogeneous transparent solution. This solution was heated at 333K until forming viscous solution and raising temperature of heating (443 K ) to generate a gel. This formed gel was fired at higher temperature of 873K for 5 h to remove the organic precursor and forming the required compound. The obtained powder wsa grinded and calcinated in air at 1473K for 10 h and coolel down in furnace cooling. This material was finally fired in the presence of oxygen gas at 1073K for 8 h to give the required La0.67Ba0.33MnO3. Different annealing conditions were performed at 1023–1223K in N2 atmosphere in order to get different oxygen stoichiometry.
In Ref.,26 Cherif et al., used sol gel method to prepare pure and homogenous La1.4(Sr1−xBax)1.6Mn2O7 (0 < x < 0.6). Suitable stoichiometric amounts of pure oxides of La, Sr, Ba, and Mn dissolved in nitric acidand the obtained solution added to citric acid with the proper molar ratio’s adding some ethylene glycol to get complete homogenous transparent solution. The solution was heated and brown gel was appeared. The formed gel was dried at temperature of 300°C, and to remove the organic matters this heating process follwed by another heating at 600 ◦C. The regrinding of the obtained black powder was done and cold pressed at 5 kbar to be finally sintered at 1000◦C for 24 h. The sol–gel method is applied in ref28 to prepare both of the La0.8Sr0.2MnO3 and Nd1-xSrxMnO3 polycrystalline manganite and they studied the influence of calcination temperature on the grain sizes of nanocrystalline sample which was found to be in the rage from 23 up to 63nm, respectively. The (La0.7Sr0.3)0.9Mn1.1O3 nanoparticles are synthesized by Dyakonov et al.,29 using coprecipitation method and thermally treated at different temperatures (800, 900, and 950°C). La1−xCaxMnO3 (x=0.33 and 0.28) powders were synthesized by both co- precipitation and sol–gel methods.30 Ohayaona reported, in ref.31 that they used a novel method to prepare nanoparticles of perovskite La0.7Ca0.11Sr0.19MnO3 based on the microwave radiation in the presence of the argon gas in a nonaqueous solvent of benzyl alcohol.
Influence of Dy addition to Co–Zn nanocrystalline spinel ferrite on the structural and magnetic properties was investigated by Urcia-Romero et al.,32 that may open the door for the possible magnetocaloric use of these spinel structure. Dy-doped nanocrystalline cobalt-zinc ferrite Co0.7Zn0.3Fe2O4 have been prepared using co-precipitation method. The modified approach allows controlling the crystalline nano-size of ferrite crystal, which enable the controle in thier magnetic properties. Furthermore different spinels are studied for example chromite spinels (MCr2O4 , where M = Co, Mn, and Ni) of homogeneous crystallinty hydrothermally prepared by reaction of different nitrates; Ni, Co, Mn nitrate as well as chromium nitrate, and sodium hydroxide in proper ratio’s to attain pH in the range of 10.5-11.5 at temperature between 180-200 ◦C for 11-13 h as shown in ref.,33
Structure of Mc Materials
According to Wang,20 CeSi alloy is crystalline in a single phase of orthorhombic crystal system of FeB-type structure. They formed with the space group NO. 62 (pnma) in corresponding to what is reported before.34,35 The determined lattice parameters a, b and c from X-ray diffraction XRD are 8.305, 3.966 and 5.963 Å, respectively,34,35 From XRD measurements performed in ref,23 the Rietveld analysis of DyCuSi showed that the formation of hexagonal single phase of Ni2In-type structure with P63/mmc space group. The lattice parameters of DyCuSi are found to be a=4.136 (5) and c=7.395 (3) Å. The measured x-ray diffraction patterns at room temperature of the Mn1.35Fe0.65P1-xSix compounds are shown in ref.34 and from XRD pattern the hexagonal Fe2P-type structure is indexed with the P–62m space group. It is quite clear that the position of the manganese atoms inside the unit cell is in 3g sites while the iron atoms is in the 3f sites, on the other hand both of the P and Si atoms have randomly postions of the 1b and 2c sites. All the lattice parameters are listed in ref.34 for all concentrations x.
The TbCo2 and Er0.2 Tb0.8Co2 alloys have cubic MgCu2 type crystal structure as shown in ref.22 Gadolinium crystal structure is hexagonal close-packed (hcp) of space group of P63/mmc (S.G No 194) with cell parameters: a = 3.636 Å, b= 3.636 Å, c = 5.7826 Å, α =90.000°, β = 90.000°, γ =120.000°.35
Figure 4: Crystal structure of Gadolinium35
Eu0.65 Sr0.35 Mn1-xFexO3 has the orthorhombic crystal structure, where A-site cations (Sr/Eu) ccupy 4c Wyckoff position (x, y, 0.25) and B-site cations (Mn/Fe) occupy 4b Wyckoff position (0.5, 0.0, 0.0). Oxygen atoms O1 and O2 in this structure occupy 4c and 4d Wyckoff positions, respectively.36 Zhong et al.,25 measured XRD for La0.67Ba0.33MnO3-d material which showed cubic perovskite structure in addition to the effect of the oxygen stoichiometry and its role in the lattice parameters. They observed that there is difference in the lattice parameters as a result of the deficiency of oxygen in La0.67Ba0.33MnO3-d and it becomes larger than the stoichiometric La0.67Ba0.33MnO3. When d increases, the lattice parameter a increase monotonously from a = 3:892 Å (d=0) to a = 3:909 Å, respectively. Sm0.6Sr0.4MnO3 is formed in the orthorhombic crystal system with space group Pnma as reported by I. A. Abdel-Latif et al.,37,38 The tilt angle of oxygen atoms in this compound plays important role in CMR magnetoresistance property.
Figure 5: Crystal structure representation of Sm0.6Sr0.4MnO3.38
As it is well-known, the rare earth manganese oxides RyA1-yMO3 crystallize either with cubic, orthorhombic, rhombohedral or hexagonal crystal system of the perovskite-like structure.39 There is a possibility that these materials crystallize with the hexagonal structure of space group P63cm when with relatively smaller ionic radius and the hexagonal phase is stable. Further attention originted from unusual magnetic order of these hexagonal manganites where Mn ions are in trivalent states with S = 2 and they are surrounded by Oxygen atoms, forming triangular bipyramids. This hexagonal manganites have both ferroelectric and antiferromagnetic properties in the same time. The (La0.7Sr0.3)0.9Mn1.1O3 nanoparticles are crystallized in the rhombohedral distortion form of perovskite-like structure of the (R3¯c) space group.40
Characterization and application of MC Materials
Cubic inter-metallic RCo2 compounds (where R is rare earth element) are exchange-enhanced Pauli paramagnet with a first-order metal-magnetic transition with application of magnetic field above a critical value observed in ErCo2 at Tc = 32 K.41 The second-order transition was observed in TbCo2 at Tc =227 K.41 The results of investigation of magneto-caloric effect in the polycrystalline Er1_xTbxCo2 (x = 1 and 0.8) are favorable and comparable with other magnetic materials with huge magneto-caloric effect of expensive and difficult to prepare them. The addition of Er to Er1_xTbxCo2 alloy does not affect the magnetocaloric potential, but affect the displacement of the magnetic transition temperature depending on the concentration of Er.42 Gd alloys; GdDy, GdTb, etc. are of the most promising candidates that show a large entropy change so we can say gadolinium and its alloys are the optimium materials up till now for the magnetic cooling use near room temperature.43
The adiabatic temperature in Gd is 3.6 K at an applied field equals to 1 T and with a Curie temperature of 293 K.44 The effect of the As concentration in MnFeP1–xAsx system showed a wonderful dependence of magnetic properties on the crystal structures.43 The crystal structure of As contents (x < 0.15), is orthorhombic while the tetragonal Fe2As-type structure is settled at higher concentration of As (x > 0.66) and these concentration represent nonmagnetic phase.45 Crystal structure transition to hexagonal Fe2P-type structure is observed in the region 0.15 £ x £ 0.66. In the ferromagnetic ordering, the magnetic moments of magnetic atoms, such as Mn and Fe, are in the same direction. The Mn ions have the divalent states and the Fe ions have a mixture of the trivalent and the divalent states. As reported in ref.,46 the fundamental components of magnetic cooling are the magnetic field generator and the magneto-caloric material and in this paper a new and original magnetic cooling technique depends on a simple principle of magnetism called the Halbach effect is described. The design and modeling presented in this work have been achieved based on the finite elements method. The first-order transition in heavy rare earth metals Dy, Tb, Er and Dy0.5Tb0.5 alloys and in Fe0.49Rh0.51 and Gd5Si1.7Ge2.3 is studied in ref.46 Some calculation of MCE such as (exchange, magnetoelastic, anisotropic and magnetic energy) were done and compared with the experimental data.47 Peltier sensor calorimetry was used to measure the entropy dependence on the magnetic field S(Ha,T ) in ref.48 The experimental setups were given in details by Basso.49 The heat flux qs is formed as a result either of a temperature change (temperature scan experiments) or of a magnetic field change (isothermal experiments) and thus one can get specific heat according to the relation;
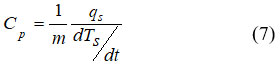
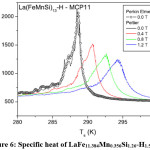
The curves of the isothermal measurements were carried out under constant field to deduce the shift in vertical entropy.48 Specific heat of LaFe11.384Mn0.356Si1.26-H1.52 were measured using Peltier calorimetry, as shown in Fig.6.
Figure 6: Specific heat of LaFe11.384Mn0.356Si1.26-H1.52b48
The ferromagnetic perovskite manganites are presented as a new class of magnetocaloric materials according to Dhahri et al.,50 They studied in details in ref50 the nature of these materials in terms of their magnetocaloric properties and the obtained results are discussed systematically. The room temperature magnetic refrigeration is one of the particular interests for energy saving and clean environmental concerns.51 Materials for magnetic cooling use depend on the magneto caloric effect; the adiabatically change of temperature of an isolated system as a result of the difference in the magnetic field.52 To design new magnetic cooling system, a great efforts have been achieved searching the so effective magneto refrigerant candidates for expanding the working temperature range towards high temperature or room temperature.53-56 For La0.6Ca0.4MnO3 in ref.,57magnetocaloric effect has been investigated and they found DSM= 5.15 J kg−1 K−1 @ H= 3 T which is about 80% of that of pure gadolinium metal.58 Practically, this promising result is important because it clear that manganites of perovskites structure is good candidates for magnetic cooling applications. The deficiency of oxygen plays an important role in the magnetocaloric effect in polycrystalline perovskites La2/3Ba1/3MnO3-d according to Zhong et al.,59 where they prepared these material by a modified sol–gel technique. The studied Curie temperatures and magnetocaloric effect show the Curie temperature dependence on the oxygen stoichiometry. The maximum change in magnetic entropy of La2/3Ba1/3MnO3 upon 10 kOe applied field was given to be DSM = 2.7 JKg-1 K-1. The characteristic data of several typical magnetic materials for magnetic refrigeration use, shown in table 1, are given as example for typical progress in this application, where it is quite clear that there is achived resonable progress. Different La manganites compared with other coumpounds. From this comparison looking at the magnetic entropy and the critical temperature one can say there are great and continuous efforts objected to get large (reasonable) magnetic entropy near room temperature.
Table 1: Typical characterstics and magnetic parameters of magnetic cooling materials
|
Sample
|
Tc (K)
|
-DSmax (JKg-1 K-1)
|
DH (T)
|
Reference of data
|
|
ErFe0.5Mn0.5O3
La0.8Ca0.2MnO3
|
9
230
|
13.5
5.5
|
7
1.5
|
Ref 60
Ref 61
|
|
La0.67Ca0.33MnO3
|
267
|
6.4
|
3
|
Ref 62
|
|
La0.6Ca0.4MnO3
La0.67Sr0.33Mn0.95V0.05O3
La0.6Pr0.1Sr0.3Mn0.9Fe0.1O3
La0.8Ba0.1Ca0.1Mn0.85 Co0.15O3
|
263
326
205
212
|
5.0
1.44
1.15
2.27
|
3
1
2
5
|
Ref 63
64
65
66
|
|
La0.65Ca0.35Mn0.9Ti0.1 O3
|
103
|
1.3
|
3
|
Ref
|
|
La0.67Sr0.33Mn0.9Cr0.1 O3
|
328
|
5.0
|
5
|
Ref 67
|
|
La0.87Sr0.13MnO3
|
197
|
7.5
|
8
|
Ref 8
|
|
La0.84Sr0.16MnO3
|
244
|
7.9
|
8
|
Ref 8
|
|
Gd
|
294
|
4.2
|
1.5
|
Ref 61
|
|
Gd0.73Dy0.27
|
265
|
10
|
5
|
Ref 68
|
|
Gd5(Si2Ge2)
|
276
|
14
|
2
|
Ref 69
|
|
Gd5(Si2Ge2)
Fe-Cr-Mn
Nd5Si4
|
300
843
75
|
7
0.37
8.3
|
5
2
5
|
Ref 69
Ref 70
Ref 71
|
Different chemical formula are suggested to obtain large magnetic entropy change (DSM~2.26 JKg-1 K-1) at Tc=354K in La0.6Sr0.2Ba0.2MnO3 by Mahn-Huong Phan et al.,72 in 0 up to 10KOe magnetic field range. According to N. H. Luong et al.,73 a large magnetocaloric effect in perovskites (La1-xNdx)0.7Sr0.3MnO3 at room temperature was found. On the other side, magnetocaloric effect found by Chen et al.,74 in Nd doped perovskite La0.7- xNdxBa0.3MnO3 polycrystalline near room temperature was discuused. Contiouns efforts still is going on and large magnetic entropy DSm was reported in ref.,73 for investigated manganese perovskites near 300K. The manganese perovskites in this work75 showed large magnetic entropy changes derived by comparable low magnetic field difference and this open the door for the household use of active magnetic refrigerant (AMR) materials. The intensive caloric effect and the difference between relative cooling powers (RCP) of La0.7Pb0.3MnO3 and 0.85(La0.7Pb0.3MnO3)–0.15(PbTiO3) composites are compared. They reported that there is an intrinsic pressure in promoting caloric efficiency in such compouns due to the elastic coupling founded between the grains.76-77
In term of magnetocaloric materials, the mixed valance manganite perovskites78 could be applied as a magnetic cooling devices. The significant large magnetocaloric effect appeared and its relation with the isothermal field-induced entropy change beyond the spin-multiplicity limit influence on some indistinctness regarding the applicability of fundamental thermodynamics. This reflect the importance of the subject undertalking and we found great number of research in the literature devoted to this field of work. Those misleading explanation concerning, for example, the difficulty of phenomenological thermodynamics are reported by Mukherjee et. al.,79 They showed that the Maxwell relation combine contributions from the following parameters; spin degrees of freedom and potential lattice degrees of freedom and their effect on the isothermal entropy change. Ising spins and pairs of exchange –coupled are studied on the base of minimalist model. The lattice degree of freedom is motivated by applied magnetic fields that allow the integrated Maxwell relation include this lattice contribution. Simply we can express realtion for the isothermal entropy change affected by application of manetic field to activiate the lattice degree of freedom. The electrocaloric effects occure with the transition from the ferroelectric to paraelectric state are discussed in details in ref80 within the Landau–Devonshire theory. In this work they found that the change from just changing the first-order phase transition into the second-order phase transition decreases the following parameters the isothermal entropy change DSM as well as the adiabatic temperature change DTad and refrigerant capacity dM. The second-order transition isothermal entropy change is reduced to one half of the first-order one, which experimently proved and is in good agreement with the magnetocaloric counterpart.
Figure 7: (a) A block of Pr0.65Sr0.35MnO3 (b) Oxide plates with their dimensions after cutting.79
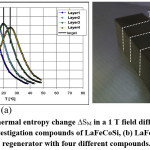
Understanding the heat transfer, which occur in the regenerator and the contribution of the intrinsic properties of the magneto-caloric material will help us to improve the efficiency of an active magnetic regenerative system.81 Legait et al compared and tested three different oxide and alloy compounds; (Pr0.65Sr0.35MnO3, La(FeCo)13-xSix, and gadolinium) applying magnetic field using a permanent magnet. They concluded that even with a low dTad, the perovskite oxide Pr0.65Sr0.35MnO3 can show significant results and they founded efficiency of the layered regenerator is better than the single layer. Moreover, a great temperature span at high frequency is obtained for high thermal conductivity material. The shape of sample they used in this work and isothermal entropy change DSM, in a 1 T field, of LaFeCoSi are shown in Fig. 7 and Fig. 8.
Figure 8: (a) Isothermal entropy change DSM in a 1 T field difference of the under-investigation compounds of LaFeCoSi, (b) LaFeCoSi regenerator with four different compounds.82
Looking at the recent research one can found that there is ongoing work on developing new materials that are both cost effective and operate in the temperature range of normal refrigeration. A giant MCE magnetocaloric effect found as a result to the deficiency of sodium in a lanthanum manganites polycrystalline is recently reported by Wali et al.,82 where they reported that there is possibity for tunning the temperature near room temperature according to the amount of deficiency of sodium in the compound. The most important results in this work are that the transition from FM into PM is in term of the second-order phase transition and occurred at the Curie temperature (Tc) , which decreased from 335 to 260 K when the increasing of the sodium deficiency rate. The change in the magnetic entropy raised from 2.38 J kg−1 K−1 to 3.48 J kg−1 K−1 by applying 2 T magnetic field and by inceasing concentation x from 0.00 to 0.15. Comparing the values obtained by Wali et al.,82 and the values reported before underlines the advantages of using the suggested oxide material for magnetic cooling application.
Magnetic propeteies, specific heat charcterstics and magnetocaloric effect investigations achived by Kalipada Das, , el al.,83 for Ln0.5Ca0.5MnO3 (Ln=Gd, Dy) rare earth calcium manganites. They found that the fairly large isothermal magnetic entropy change at low temperature that may be interpreted in terms of the effect of the magnetic rare-earth precursor ions. The impact of the disorder oon the magnetocaloric effect in Ti doped manganites was investigated by El. Kossi et al.,84 where they found that the magnetic entropy change strongly depends on the Ti concentration. The most promising results are observed by I. A. Abdel-Latif et al.,85 where the Nd 0.6 Sr 0.4 MnO3 nanocomposites showed MR of 99.84% at room temperature that enable us to industially apply these materials for magnetic devices fabrication.
Theoretical calculations will help us to design new materials with desired properties. Spin-dimer systems are rich applicable systems for quantum phase transitions studies tunned by magnetic field. According to the study of Straßel.86 There is possibility to It is possible to notice the shift from the characteristic scale closed to the critical points to the behavior of a definite-temperature phase transition. They studied two-dimensional coupled spin-dimer systems. Moreover, they used the the magnetization dependence temperature descriping the magnetocaloric effect to define the accurate critical field. Gharsallah et. Al. studied the magneto caloric effect for La0.6Sr0.4MnO3 and La0.6Ca0.4MnO3 using theoretical calculations87 which showed large and tunable suggesting their possible applications in moderate magnetic field and near room temperature.
M. A. Hamad88 has invented a phenomenological model to simulate the dependence of the magnetization upon the variation in temperature for the magnetocaloric materials, such as, La0.67Ca0.33MnO3,89 Ce0.67Sr0.33MnO3,88 La0.75Ca0.25MnO3.91 According to I. A. Abdel-Latif et al., [92-93], the calculated ground state of magnetization value in Eu0.65Sr0.35FexMn1-xO3 and its decrease as a result of the Fe-doping increase which may be attributed to the increase in the spin-down with the increase in the Fe-doping. E. P. Nobrega et al.,94 discussed the magnetocaloric effect in metallic Gadolinium using Hamiltonian 4f-spins model and considered the 4f spin-spin interaction based on the mean field approximation as well as the Monte Carlo simulation. Their calculation showed that reasonable results for the magnetocaloric potentials DSM and DTad according to the mean field approximation but somehow there is failure in interpretation of the experimental specific heat data at the magnetic ordering temperature. Also Monte Calro method was used95 to calculate the magnetocaloric effect of the second order the magnetic phase transition in Gd5(SixGe1−x)4 for x>0.5 compounds. They showed the good agreement between the experimental isothermal entropy change and the experimental adiabatic temperature change upon magnetic field variations and their theoretical calculaions. The Potts-like model (Monte Carlo method) is used to define the magnetic and magnetocaloric properties in Gd1xCx (with x= 0.25 0, 0.025, 0.06, 0.09) alloy.96 The decrease in the magnetization at low temperature was found but on the other hand FM – PM phase transition temperature increases, as a result of the increase in the C-atom concentration in the system. The isothermal magnetic entropy variation and the refrigerant capacity in binary alloy is comparable higher than in pure Gd material. It is quite clear that still great interest and effort that are devoted to studying the magnetocaloric effect in order to develop clean, cheap and environmentally friend refrigerator technology. The main challenges in the current magnetic cooling is that more efficient cooling system needs high magnetic field which is out of use in the industrial scale and very low temperature. So this work is a try Much more efforts were carried out to develop magnetic refrigerator materials with reasonable conditions for environmentally friend refrigeration based on the success observed in the of our recent works85 and.82-83 Recently, Pramanick et al.,97 observed multiple magnetic transition points as well as large magnetocaloric effect and magnetoresistance at room temperature in Nickel FM alloy (Ni2.048Mn1.312In0.64). Tetranuclear rare earth (RE) complexes [RE4(acac)4L6(μ3-OH)2] (HL = 5-(4-fluorobenzylidene)-8-hydroxylquinoline; acac = acetylacetonate; RE = Y (1), Eu (2), Gd (3), Tb (4), Dy (5), Tm (6) and Lu (7)) have been introduced in ref.,98 and the found magnetocaloric effect (MCE) was ΔSM(T) = 20.8 J kg−1 K−1 for complex 3 where R.E. is Gd. According to Makni-Chakroun et al.,99 the shift in maximum magnetic entropy change was one percent higher than of both calcium and lanthanum deficiencies in La0.7Ca0.3MnO3 (LCMO) while the reduce in both the relative cooling power (RCP) and the full-width at half-maximum values were observed. Local magnetic behavior across the first order phase transition in magnetocaloric compound; LaFeCoSi was studied using magneto optical imaging technique.100 The size and shape of the sample as well as the structural defects or micro-cracks are very important parameters. Large magnetocaloric effect in single crystal form of the RFeO3 (R = Tb or Tm) was observed in ref101 by the rotation occurred in the magnetization with strong magneto crystalline anisotropy. The charge distribution difference of 4f-electrons between Tb3+ and Tm3+ ions may lead to the different signs field entropy rotatation; −ΔSM = 17.42 J kg−1 K−1, and –ΔSM = −9.01 J kg−1 K−1 @ 9 K and 17 K in the field difference 50 kOe for TbFeO3 and TmFeO3 single crystals, respectively from b axis to c axis.102 Furthermore, in ref.102 Nd0.6Sr0.4MnxCo1-xO3 nanocomposites experiencing different strength magnetic fields were studied where, the the temperature dependence of the magnetic entropy change was found. The highest negative magnetic entropy value (6.46 J/kg K) was represented around Curie temperature for the sample of x=0.7 moreover, with comparable RCP (333.16 J.Kg-1 at 3T and 3007.14 Jkg-1 at 9 T ). These results are promising and showed how pregress is achived in these materials.
According to Felhi et al.,103 the magnetocaloric effect was found in the spinel ferrites oxides such as Zn0.7Ni0.3-xCuxFe2O4 prepared using the sol-gel technique. They found that monotonically decreasing in Curie temperature TC from 327k as a result of the partial substitution of Ni. The obtained magnetic entropy change values at 2 T ; ∆SMmax for x = 0, 0.1 and x =0.2 were 0.67, 0.64 and 0.62 J/kgK, respectively, and the obtained relative cooling power (RCP) were 111, 117 and 124 J/kg , respectively at the same magnetic field. According to RCP values they found, one can say these materials are promising candidates for magnetic refirigration use.
Large magnetocaloric effect in YbPt2Sn as well as the adiabatic demagnetization refrigeration was reported by Jang et al.,104 where they found the weak magnetic coupling occurred between Yb atoms, that may lead to preventing them from ordering in very low temperature above 0.25 K. This may leave enough entropy free for use in adiabatic demagnetization cooling. This large capacity in the volumetric entropy in YbPt2Sn compound is enough for a good to obtain considerable cooling power.101 Recently, it is reported103 in details about two successive first-order transitions in Mn1-xFexNiGe alloys; including a martensitic transition (MT) and an antiferromagnetic-ferromagnetic (AFM-FM) transition. The first-order magnetoelastic nature was clear, according to the Arrott plot. On the other hand, the performances of Mn1-xFexNiGe in this work as magnetocaloric and negative thermal expansion materials were studied where the impressive value of isothermal entropy change reached −25.8 J/kg K @ 203 K for x = 0.11 comparing with the other two compounds. The observed giant negative thermal expansion and near-zero thermal expansion for different temperature ranges were found in the Fe-doped samples.105 A series of the Gd5.1Si2Ge2−xBx alloys synthesized by Xue-ling HOU, et al.,106 based on the arc melting method in purified argon gas and they described the influence of boron addition on the magnetocaloric effect. The results showed a small amount of B does not change monoclinic structure of the Gd5Si2Ge2 composite with superior magnetocaloric effect. The maximum change in the magnetic entropy (|∆SM|) of x=0.01 sample was found to be 13.7 J/(kg·K) in 1.5 T magnetic field and at 288 K. Comparing with table 1 one can note that is promising materials for magnetic refrigeration.
Acknowledgements and Funding Source
The author is thankful to the Deanship of Scientific Research in Najran University for their financial support NU/ESCI/16/063 in the frame of the local scientific research program support.
Conclusions
- It is clear that theoretical and experimental efforts are going to develop new materials for magnetic refrigeration applications as green and friendly environmentally technology to reduce the pollution.
- The achieved scientific efforts to find optimum and promising properties for the magnetic refrigeration application are great and there are reasonable progress because of the promising results achieved in this field.
- The magnetic refrigerator has higher cooling efficiency than conventional vapor refrigerator CVM where its cooling efficiency ~30-60% while the cooling efficiency in CVM ~ 5-10% and this open the door for powerful materials for clean and safe refirigration use.
- The low coast and small size of magnetic cooling is one the important advantage.
- Large magnetic entropy changes derived by comparable low magnetic field difference may open the door for the household use of active magnetic refrigerant (AMR) materials
References
- http://en.wikipedia.org/wiki/Magnetic_refrigeration
- Goodenough J. B., Phys. Rev. 1955, 100, 564.
- V.K. Pecharsky, K.A. Gschneidner, A.O.,Tsokol, Rep. Prog. Phys. , 2005, 68, 1479
- H. Zhu, H. Song, Y.H. Zhang, Appl. Phys. Lett. 81 (2002) 3416
- A. H. Morrish, The Physical Principles of Magnetism(Wiley, New York, 1965, Chap.3.
- A.M.Tishin, K.A.Gschneidner, Jr.,and V.K.Pecharsky, Phys.Rev.B59, (1999) 503.
- Z.B. Guo, Y.W. Du, J.S. Zhu, H. Huang, W.P. Ding, D. Feng, Phys. Rev. Lett. 78 (1997) 1142.
- Manh-Huong Phan, Seong-Cho Yu, Journal of Magnetism and Magnetic Materials 308 (2007) 325.
- E. Bruck, J. Phys. D: Appl. Phys. 38 (2005) R381.
- E. Warburg, Ann. Phys. 13 (1881) 141.
- V.K. Pecharsky, K.A. Gschneidner, Phys. Rev. Lett. 78 (1997) 4494.
- X. Hu, B.G. Shen, J.R. Sun, G.H. Wu, Phys. Rev. B 64 (2001) 132412.
- H. Wada, Y. Tanabe, Appl. Phys. Lett. 79 (2001) 3302.
- S. Fujieda, A. Fujita, K. Fukamichi, Appl. Phys. Lett. 81 (2002) 1276.
- Q. Tegus, E. Bruck, K.H. Buschow, F.R. de Boer, Nature 415 (2002) 150.
- M.H. Phan, S.C. Yu, N.H. Hur, Appl. Phys. Lett. 86 (2005) 072504.,
- Zhao-Jun Mo, Jun Shen, Li-Qin Yan, Cheng-Chun Tang, Jing Lin, Jian-Feng Wu, Ji-Rong Sun, Li-Chen Wang, Xin-Qi Zheng and Bao-Gen Shen, Appl. Phys. Lett., 103 (2013) 052409 http://dx.doi.org/10.1063/1.4816729.
- Emsley, John (2001). Nature’s Building Blocks. Oxford University Press. p. 342. ISBN 0-19-850341-5.
- V.K. Pecharsky, K.A. Gschneidner, Annu. Rev. Mater. Sci. 30 (2000) 387.
- L.C. Wang, Q.Y. Dong, J. Lu, X.P. Shao , Z.J. Mo, Z.Y. Xu , J.R. Sun, F.X. Hu, B.G. Shen, Journal of Alloys and Compounds 587 (2014) 10.
- M. F. Md Din, J. L. Wang, S. J. Campbell, A. J. Studer, M. Avdeev, S. J. Kennedy,Q. F. Gu, R. Zeng, and S. X. Dou, Applied Physics Letters 104, (2014) 042401
- J. Chen, B.G. Shen, Q.Y. Dong, J.R. Sun, Solid State Communications 150 (2010) 1429.
- A.M. Gomes et al., Journal of Magnetism and Magnetic Materials 242–245 (2002) 870.
- M.H. Phan, S.B. Tian, D.Q. Hoang, S.C. Yu, C. Nguyen, A.N. Ulyanov, Journal of Magnetism and Magnetic Materials 258–259 (2003) 309.
- W. Zhong et al., Journal of Magnetism and Magnetic Materials 261 (2003) 238.
- K. Cherif et. al., Journal of Alloys and Compounds , 2007, 432, 30.
- J. Ma, M. Theingi, Q. Chen, W. Wang, X. Liu, H. Zhang, Ceramics International 39, 7, (2013) 7839.
- I. A. Abdel-Latif et. al., Scientific Reports, 2019, https://doi.org/10.1038/s41598-019-50393-9.
- V. Dyakonov, A. Slawska-Waniewska, J. Kazmierczak, K. Piotrowski, O. Iesenchuk, H. Szymczak, E. Zubov, S. Myronova, V. Pashchenko, A. Pashchenko, A. Shemjakov, V. Varyukhin, S. Prilipko, V. Mikhaylov, Z. Kravchenko, A. Szytu³a and W. Bazela, Low Temp. Phys. 35, (2009) 568.
- Peka M.; Drozd V., Journal of Non-Crystalline Solids, 2008, 354, 5308.
- E. Ohayona, A. Gedankena, Journal of Magnetism and Magnetic Materials, 331, (2013), 45.
- S. Urcia-Romero, O. Perales-Pérez and G. Gutiérrez, J. Appl. Phys. 107 (2010) 09A508
- S. K. Durrani, S. Z. Hussain1, Kh. Saeed, Y. Khan, M. Arif, N. Ahmed, Turk J Chem 36 (2012) , 111.
- G. Yao-Xiang, et al, Chin. Phys. B Vol. 21, No. 3 (2012) 037504
- http://www.webelements.com/gadolinium/crystal_structure_pdb.html and F. H. Spedding, A. H. Daane, and K. W. Herrmann, Acta Crystallogr., 9, (1956), 559.
- I.A. Abdel-Latif, S.A.,Saleh, J. Alloys Comp., 2012, 530, 116.
- I.A. Abdel-Latif et al. I.A., J. Alloys Comp., 2008, 452, 245.
- I. A., Abdel-Latif , Th M. El-Sherbini., Materials sciences Indian Journal MSAIJ, 2010 , 6(2), 109.
- I.A. Abdel-Latif , AIP Conf. Proc., 2011, 1370, 108
- F. Garcia, M.R. Soares, A.Y. Takeuchia, S.F. da Cunha, J.Alloys Comp. 279 (1998) 117.
- J. Voiron, A. Berton, J. Chaussy, Phys. Lett. 50A (1974) 17.
- V. Provenzano, A.J. Shapiro, and R.D. Shull, Nature 429 (2004) 853.
- L. T. Kuhn, N. Pryds, C R H Bahl and A Smith, J. of Phys.: Conf. Series 303 (2011) 012082.
- R Zach, M Guillot and R Fruchart J. Magn. Magn. Mater. 89 (1990) 221.
- Y Takeda, T Okane, T Ohkochi, S -i Fujimori, Y Saitoh, H Yamagami, H Yabuta and T Takabatake J. of Phys.: Conference Series 200 (2010) 012199.
- H.R.E.H. Bouchekara , A. Kedous-Lebouc, J.P. Yo, Inter. Joural of refrigeration 35 ( 2012 ) 115.
- Y.I. Spichkin , M. Tishin, Journal of Alloys and Compounds 403 (2005) 38.
- V. Basso, C. P. Sasso, M. Kupferling, Inter. Joural of refrigeration 37 ( 2014 ) 257.
- Basso et al.,. Rev. Sci. Instrum. 81 ( 2010) 113904.
- Essebti Dhahri, Moez Bejar, Safa Othmani, Anowar Tozri and El Kebir Hlil, Smart. Materials for Energy, Communications and Security, (2008) I, 31.
- Cherif et al., Journal of Alloys and Compounds 432 (2007) 30.
- V. K. Pecharsky and K. A. Gschneider, Phys. Rev. Lett. 78 (1997) 4494.
- H. Oesterreicher, F.T. Parker, J. Appl. Phys. 55 (12) (1984) 4334.
- R.D. Mc Michael, J.J. Ritter, R.D. Shull, J. Appl. Phys. 73 (1993) 6946.
- H. Takeya, V.K. Pecharsky, K.A. Gschneidner Jr., J.O. Morman, Appl. Phys. Lett. 64 (1994) 2739.
- H. Zhu, H. Song, Y.H. Zhang, Appl. Phys. Lett. 81 (2002) 3416
- Z.B. Guo, Y.W. Du, J.S. Zhu, H. Huang, W.P. Ding, D. Feng, Phys. Rev. Lett. 78 (1997) 1142.
- X. Bohigas, J. Tejada, M.L. Marinez-Sarrion, S. Tripp, R. Black, Journal of Magnetism and Magnetic Materials 208 (2000) 85.
- W. Zhong, W. Chen, C.T. Au, Y.W. Du, Journal of Magnetism and Magnetic Materials 261 (2003) 238.
- K. Chandran, P.N. Lekshmi, P.N. Santhosh, High temperature spin reorientation, magnetization reversal and magnetocaloric effect in 50% Mn substituted polycrystalline ErFeO3, Journal of Solid State Chemistry (2019), doi: https://doi.org/10.1016/j.jssc.2019.120910.
- Z.B. Guo, Y.W. Du, J.S. Zhu, H. Huang, W.P. Ding, D. Feng, Phys. Rev. Lett. 78 (1997) 1142.
- Young Sun, X. Xu, Yuheng Zhang, Journal of Magnetism and Magnetic Materials, 219 (2000) 183.
- X. Bohigas, J. Tejada, E. Del Barco, X.X. Zhang, M. Sales, Appl. Phys. Lett. 73 (1998) 390.
- S. Mnefguietal. et. al., Journal of Solid State Chemistry, 215 (2014) 193–200.
- R. Cherifetal., et. al., Journal of Solid State Chemistry, 215 (2014) 271–276.
- M.A. Gdaiem et al., Materials Research Bulletin 88 (2017) 91–97
- Young Sun, Wei Tong, Yuheng Zhang, Journal of Magnetism and Magnetic Materials 232 (2001) 205.
- V.K. Pecharsky, K.A. Gschneidner, Appl. Phys. Lett. 70 (1997) 3299.
- K.A. Gschneidner Jr., V.K. Pecharsky, J. Appl. Phys. 85 (1999) 5365.
- X. Hai et al., Journal of Solid State Chemistry 277 (2019) 680–685.
- L.S. Paixão, et al., Journal of Magnetism and Magnetic Materials 493 (2020) 165693.
- Mahn-Huong Phan, Seong-Cho Yu, A. N. Ulyanov, M. K. Lachowicz, Material Science Vol 21 No 1 (2003) 133.
- N. H. Luong, N. T. M. Phuong, P. T. Hien, H. N. Nhat, L. H. Hoang, N. Chau, N. H. Hai, VNU Journal of Science, Mathematics – Physics 24 (2008) 30.
- W. Chen, L.Y. Nie, W. Zhong, Y.J. Shi, J.J. Hu, A.J. Li, Y.W. Du, J. Alloys. Compd. 395 (2005) 23.
- Manh-Huong Phan, Hua-Xin Peng, Seong-Cho Yu, N. D. Tho, H. N. Nhat, N. Chau, Journal of Magnetism and Magnetic Materials 316 (2007) e562.
- E. Mikhaleva, E. Eremin, I. Flerov, A. Kartashev, Journal of Materials Research, 30, ( 2015), 278-285 DOI: https://doi.org/10.1557/jmr.2014.369.
- E. Mikhaleva, I. Flerov, A. Kartashev, M. Gorev, Journal of Materials Research 28 (2013) , pp. 3322-3331 DOI: https://doi.org/10.1557/jmr.2013.360
- C. R. H. Bahl, D. Velázquez, K. K. Nielsen, K. Engelbrecht, K. B. Andersen, R. Bulatova, N. Pryds, Appl. Phys. Lett. 100 (2012) 121905.
- T. Mukherjee, S. Michalski, R. Skomski, D. J. Sellmyer, and Ch. Binek, Phys. Rev. B 83 (2011) 214413.
- B. Li, W. J. Ren, X. W. Wang, H. Meng, X. G. Liu, Z. J. Wang, and Z. D. Zhang Appl. Phys. Lett. 96 (2010) 102903.
- U. Legait, F. Guillou, A. Kedous-Lebouc , V. Hardyb, M. Almanza, Inter. Joural of refrigeration 37 ( 2014 ) 147.
- M. Wali, R. Skini, M. Khlifi, E. Dhahri and E. K. Hlil, Dalton Trans., 44 (2015), 12796-12803.
- Kalipada Das, Tapas Paramanik, I. Das, Journal of Magnetism and Magnetic Materials 374 (2015) 707–710.
- S. El. Kossy, S. Ghadhbane, S. Mnefigue, E. Dhahri and E. K. Hlil, Journal of Magnetism and Magnetic Materials 395 (2015) 134–142
- I. A. Abdel-Latif, Adel A. Ismail, H. Bouzid , A. Al-Hajry, Journal of Magnetism and Magnetic Materials 293 (2015) 233.
- Dominik Straßel, Peter Kopietz, and Sebastian Eggert, Phys. Rev. B 91 (2015) 134406.
- H. Gharsallah, M. Bejar, E. Dhahri and E. K. Hlil, L. Bessias, Ceramic International, (2015) doi:10.1016/j.ceramint.2015.08.167.
- M. A. Hamad, Phase Transitions, 85 (2012) 106–112.
- M. A. Hamad, J. Therm Anal Calorim 111 (2013) 1251–1254
- M. A. Hamad, J. Supercond Nov. Magn., 26, Issue 9, (2013)2981-2984.
- M. A. Hamad , Journal of Advanced Ceramics 1(4) 2012, 290-295.
- I. A. Abdel-Latif, M. R. Ahmed, I. A. Al-Omari, A Sellai, Journal of Magnetism and Magnetic Materials, 420 (2016) 363–370.
- I. A. Abdel-latif, IOP Conf. Series: Materials Science and Engineering 146 (2016) 012003 doi:10.1088/1757-899X/146/1/012003.
- E.P. Nobrega,, N.A. de Oliveira, P.J. von Ranke, A. Troper, Journal of Magnetism and Magnetic Materials, 320 (2008) e147–e149
- E. P. Nóbrega, N. A. de Oliveira, P. J. von Ranke, and A. Troper, Phys. Rev. B 72 (2005) 134426.
- Yan Ma, An Du, Journal of Magnetism and Magnetic Materials, 321 (2009). L65–L68
- S. Pramanick, P. Dutta, S. Chatterjee, S. Giri, S. Majumdar, Journal of Magnetism and Magnetic Materials Volume 405 ( 2016) 270–273.
- Hong-Ling Gao, Li Jiang, Shuang Liu, Hai-Yun Shen, Wen-Min Wang and Jian-Zhong Cui, Dalton Trans., 45 (2016) 253.
- J. Makni-Chakroun, , H. Omrani, W. Cheikhrouhou-Koubaa, M. , A. Cheikhrouhou, Journal of Superconductivity and Novel Magnetism 2016 pp 1-9.
- C. Bennati, F. Laviano, G. Durin, E. S. Olivetti, V. Basso, G. Ghigo, M. Kuepferling, , Journal of Magnetism and Magnetic Materials Volume 400 ( 2016) 339.
- Ya-Jiao Ke, Xiang-Qun Zhang, Yue Ma & Zhao-Hua Cheng, 6, Sci. Rep. (2016) 19775; doi: 10.1038/srep19775.
- I.A. Abdel-Latif et al. , Journal of Magnetism and Magnetic Materials 457 (2018) 126–134.
- R. Felhi, H. Omrani, M. Koubaa, W.Cheikhrouhou Koubaa, A. Cheikhrouhou , Journal of Alloys and Compounds 758 (2018) 237e246.
- Jang, D. et al.. Nat. Commun. 6 (2015), 8680 doi: 10.1038/ ncomms9680 (2015)
- K. Xu, et al., Sci. Rep. 7 (2017) 41675; doi: 10.1038/srep41675.
- Xue-ling HOU, et al, Progress in Natural Science: Materials International 21(2011) 413−417.

This work is licensed under a Creative Commons Attribution 4.0 International License.
 and Mahrous R. Ahmed3
and Mahrous R. Ahmed3 Material Science Research India An International Peer Reviewed Research Journal
Material Science Research India An International Peer Reviewed Research Journal