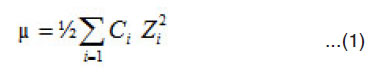Formation Constants of Lanthanide Metal Ion Chelates with Some Substituted Sulfonic Acids

R. S. Sonone* and G. H. Murhekar

Department of Chemistry, Govt. Vidarbha Institute of Science and Humanities, Amravati- 444 604, India.

DOI : http://dx.doi.org/10.13005/msri/070131

ABSTRACT:

The pKa and logK values of some substituted sulfonic acid in 70% (v/v) dioxane-water mixture have been determined using pH metric measurements. The stability constant of complexes of Gd (III), Tb (III) and Ho (III) with p-aminobenzenesulfonic (L₁) and p-sulfophthalic acid (L₂), 2-amino-5-chloro benzenesulfonic acid (L₃) and trifluoromethanesulfonic acid (L₄) have been determined at 0.1M ionic strength at 28±0.1 ⁰C pH metrically. It is observed that both the metal ions formed 1:1 & 1:2 complexes with all the ligands.

KEYWORDS: Stability constant; Chelates; Sulfonic acids

Copy the following to cite this article: Sonone R. S, Murhekar G. H. Formation Constants of Lanthanide Metal Ion Chelates with Some Substituted Sulfonic Acids. Mat.Sci.Res.India;7(1)

Copy the following to cite this URL: Sonone R. S, Murhekar G. H. Formation Constants of Lanthanide Metal Ion Chelates with Some Substituted Sulfonic Acids. Mat.Sci.Res.India;7(1). Available from: http://www.materialsciencejournal.org/?p=2303

Introduction

Substituted sulfonic acid has been use as chelate forming agent because of increased stability of these derivatives in water than parent ligand. Many substituted sulfonic acids are well know for enhance biological activities.¹ Substituted sulfonic acid are also used as anti inflammatory agent insecticides bacterial and for pharmaceutical fungicide.^2-3 Studies of Fe (III) ion complexes with 8-hydroxyquinoline 7-sulfonic acid by spectrophotometric technique have been studied.⁴ Stability constant of 8-amino1-naphthol and its different sulfonic acid derivatives have been studied.⁵

Influence of ionic strength of lanthanide metal ion complexes in ethanol-water mixture have been reported.⁶ Stability constant of lanthanide metal ions and transition metal ions with substituted pyrazoles and isoxazoles have been studied recently in our laboratory.^7-8 pH-metric studies on formation constants of the complexes of substituted pyrazoles with some lanthanide metal ions and the influence of ionic strengths on complex equilibria in a 70% dioxane-water mixture have been reported.⁹ Gupta et al have determined stability constant of transition metal ion complexes with disalicylamides at different ionic strength.¹⁰ Hence moment have come to study the proton ligand stability constant of Gd (III), Tb (III)  and Ho (III), with p-aminobenzenesulfonic acid (L₁), p-sulfophthalic acid (L₂), 2-amino-5-chlorobenzenesulphonic acid (L₃) and trifluoromethanesulfonic acid (L₄) at 0.1 M ionic strength in 70% (v/v) dioxane-water mixture at 28± 0.1 ^oC pH-metrically.

Experimental

The substituted sulfonic acids are insoluble in water, hence 70% dioxane-water mixture (v/v) was used as solvent. Lanthanide metal nitrates were dissolved in double distilled water and their concentration estimated by standard method.¹¹ Sodium hydroxide, KNO₃, and nitric acid, used were of AR grade. Dioxane was purified by standard method.¹² pH measurement were carried out with ELICO pH meter (accuracy ± 0.05 units) using combined electrode at 28 ± 0.1⁰C.

Calvin Bjerrum Titration Technique

The titrations were carried out in an inert atmosphere of nitrogen. The ionic strength of solution was maintained constant by adding an appropriate amount of 1M KNO₃ solution. The values were recorded by pH meter. These values converted to [H⁺] values by applying the correction proposed by Van Uitert & Hass.¹³

The overall 0.1 ionic strength of solution was calculated by expression

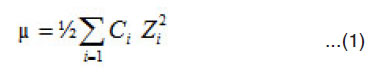
The contribution of the other ions in addition to K⁺ and NO^–₃ also taken into consideration.

Results and Discussion

The titration data were used to construct the curve between volumes of NaOH vs pH. They are called as acid titration curve, ligand titration curve and metal titration curve. The pK values of ligand and logK values of Gd (III), Tb (III) and Ho (III) complexes at 0.1 M ionic strength were calculated by Irving and Rossotti’s method.¹⁴

Proton–Ligand Stability Constants

Substituted sulfonic acids may be considered as monobasic acids having one replaceable H⁺ ion from -OH^– group and can therefore be represented as HL

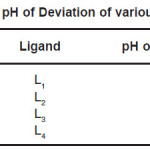
The titrations data were used to construct the curve between volumes of NaOH Vs pH. It is observed from the titration curve that the ligand curves start deviating from free acids curve at about pH 3.40 for L₁, at about pH 2.90, at about pH 3.44 for L₃ and at about pH 3.70 for L₄. The deviation increased continuously up to pH 12.50. It indicated that OH^– group start to dissociated at about 2.90 and complete its dissociation at about pH 12.50. It can be summarized in table -01.

Table 1: pH of Deviation of various ligand

Click on image to enlarge

The average number of proton associated with the ligand

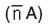
was determined from ligand titration curves employing the equation of Irving and Rossotti. The pK values were estimated from formation curves

by noting the pH, at which

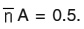
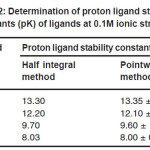
The accurate values of pK were estimated by pointwise calculations which are presented in table -02. The pK values of ligands increases in the following order.

L₁ > L₂  > L₃ > L₄

It could be seen from the values, the more reduction in pK values of ligand L₄ may be due to presence of fluoride groups which act as stronger electron withdrawing groups.

Table 2: Determination of proton ligand stability constants (pK) of ligands at 0.1M ionic strength

Click on image to enlarge

Metal–Ligand Stability Constants

Metal ligand stability constants of Gd (III), Tb (III) and Ho (III) complexes with some substituted sulfonic acid were determined by employing Calvin-Bjerrum pH-metric titration technique as adopted by Irving and Rossotti. The formation of chelates between Gd (III), Tb (III) and Ho (III) with substituted pyrazoles was indicated by

The significant departure starting from pH 2.97 for Gd (III), pH 2.91 for Tb (III)   and pH 2.89 for  Ho (III) complex system.

The change in colour from, colorless to yellow and then dark yellow as pH increased from 3.00 to 12.50.

The logK values were directly read from the formation curves

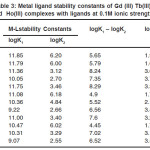
using half integral method. The most accurate logK values were calculated by pointwise calculation method are presented in table -03 for all the systems. The logK₁ and logK₂ values follow the order as

Gd (III) < Tb (III) < Ho(III)

It could be seen that logK values follow increasing trend. This is due to the electron withdrawing group (like F^–,Cl^–, Br^– and I^– ). The values of logK, (logK₁ – logK₂), and (logK₁ / logK₂) are in good agreement with excepted values. It is observed that the similar difference may be due to trans effect 

The results show that, the ratio logK₁ / logK₂ is positive in all cases. This implies that there is little or no steric hindrance to the additions of secondary ligand molecule.

Table 3: Metal ligand stability constants of Gd (III) Tb(III) and Ho(III) complexes with ligands at 0.1M ionic strength

Click on image to enlarge

Validity of LogK = a pK + b

The linear relationship logK = a pK + b has been found [15], to hold good for transition metal complex of series of closely related ligands. The stability of the metal complexes of substituted sulfonic acids follows the order,

Gd (III) < Tb (III) < Ho (III)

The plot of logK₁ Vs pK and logK₂Vs pK show satisfactory linear relationship giving slope values of 1.00 and 1.05 respectively. The partial molar free energies of metal ligand and proton ligand complexes exactly compensate with each other. When logK Vs pK plot is linear with a slope of unity. From table-03 Gd (III), Tb (III) and Ho (III) metal ions formed 1:1 and 1:2 complexes with all the ligands.

References

1. Palapova I.A., Purygin P.P, Lipatov I.S.;Pharm. Chem. J. Newyork, 35(1): 5-7 (2001).
2. Sofia Tedelind, Fredrik Westberg, Martin Kjerrulf, Alexander Vidal ; World J Gastroenterol., 28, 13(20): 2826-2832,(2007).
3. Subramanian, Sreedhar; Campbell, Barry James; Current Opinion in Infectious Diseases, 19(5): 475-484, (2006).
4. Bjerrum J., Chem. Rev; 46: 381 (1950).
5. Vartak,D. G. and Jose C.J., Ind.J.Chem, 10:635 (1972).
6. Murhekar G. H., Narwade.M.L.,Raut A.R.and Banewar V.W.; Vidarbh J.Sci. 4(3-4):67-74 (2009).
7. Murhekar G.H., Raut A.R. and Wadekar M.P.;Oriental J. Chem. 25(4): 1093-1096, (2009).
8. Pawar R.P.,Raut V.M., Murhekar G.H,; Oriental J. Chem., 25(4): 1117-1120,(2009).
9.  Naik A.B. AND Narwade M.L., Russian J.Coor. Chem,. 35(12): 932-937 (2009).
10.  Gupta A. and Pannu B.S., Asian J.Chem.,6(1): 1742 (1994).
11. Vogel A.I. A text book of quantitative inorganic analysis, logmans Green, Landon, 589(1975).
12. Vogel A.I., Text book of practical organic chemistry, 177 (1956).
13. Van L.G., Vitert and Hass C.: J Am Chem Soc. 75: 451 (1953).
14. Irving H. M. and Rossotti H.S, J Chem. Soc.,3397 (1953).
15. Hones J.G. Tomkinson J.B., Poole and Williams J.P., J. Chem Soc., 3125 (1958).

---

This work is licensed under a Creative Commons Attribution 4.0 International License.