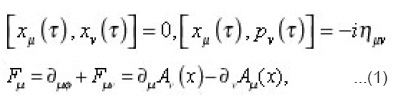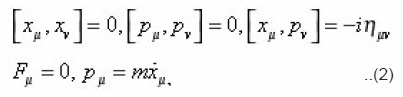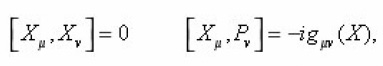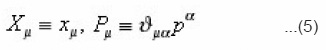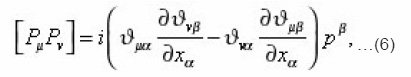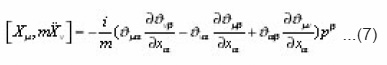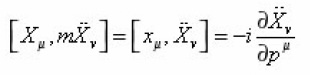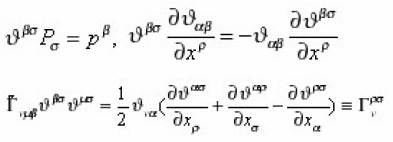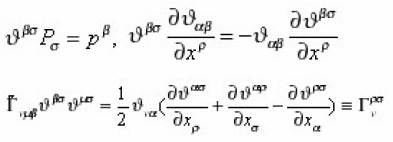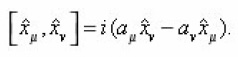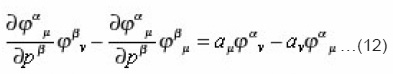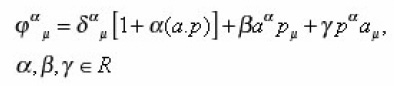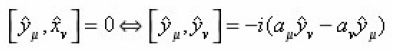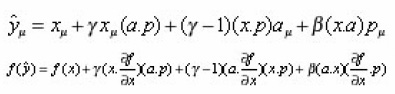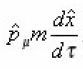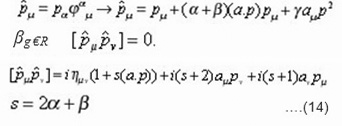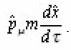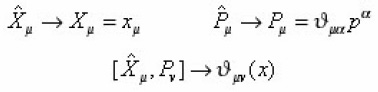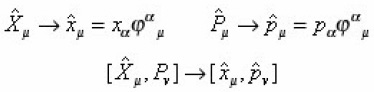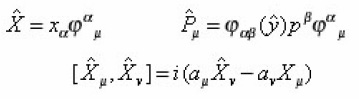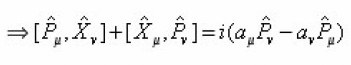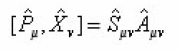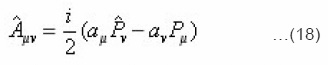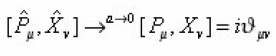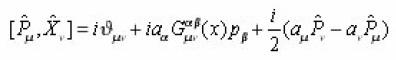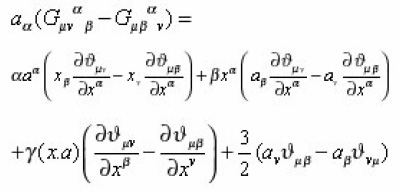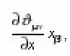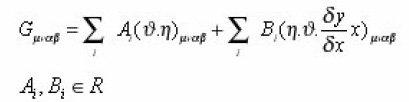PDF Downloads: 809

On Characterization of Non-Commutative Minkowski Space Time

Dharmendra Kumar¹ and Sunil KumarYadav²

¹Department of Physics, K.B. Womens College, Madhepura, India.

²Department of Physics, C.N. College, Ramgarh, India.

DOI : http://dx.doi.org/10.13005/msri/090117

Article Publishing History
Article Received on : 12 June 2012
Article Accepted on : 05 July 2012
Article Published :
Plagiarism Check: No

Article Metrics

ABSTRACT:

The present study aims to derive modified geodesic equation in non-commutative space time. Snyder developed a model for non-commutative space time which provides a suitable technique of quantum structure of the space. We extend Tetrad formulation of general relativity to non-commutative case for complex gravity models. We derive geodesic equation on the k-space time in Non-commutative space, which is a generalization of Feynman’s approach. It has been shown that the homogeneous Maxwell’s equations may be derived by starting with the Newton’s force equation and generalized to relativistic. We show that the geodesic equation in the commutative space time is a suitable for generalization to κ -space time in κ -deformed space time. It shown that the κ-dependent correction to geodesic equation is cubic in velocities.

KEYWORDS: Geodesic; Non-commutative; Space time; Newtonian; Gravity model; Feynmann; Deformed

Copy the following to cite this article:

Kumar D, Yadav S. K. On Characterization of Non-commutative Minkowski Space Time. Mat.Sci.Res.India;9(1)

Copy the following to cite this URL:

Kumar D, Yadav S. K. On Characterization of Non-commutative Minkowski Space Time. Mat.Sci.Res.India;9(1). Available from: http://www.materialsciencejournal.org/?p=1205

Introduction

Several field theory models on k- space time have been constructed , using different techniques such as by F. Mejer and J Lukierski specially in, scalar field theory on k-Minkowski space. It has been shown by S. Tanimutra that in the flat space time, Feynman approach and minimal coupling method are equivalent which is usefull to derive the general equation of motion for a charged particle. We discuss here the applicability of Feynman approach for the case of general relativity, resulting in the derivation of the geodesic equation. We generalize the procedure for k-Minkowski space. We obtain here corrections to the geodesic equation due to the k-deformation of space time , up to the first order in the deformation parameter. WE know that a relativistic particle of mass m and electric charge e is described by in 4D-Minkowski space, where xμ(τ) in 4D- Minkowski space, where τ is a parameter. Let us write the following relations

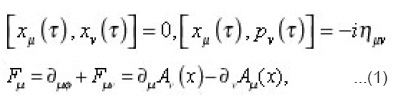
vol9_no1_cha_dha_eq1

Where p_μ= mx + eA_μis the canonical momentum operator, F_μ= mx, the force_μνthe electromagnetic strength tensor, A_μ is a gauge field and Φ(x) is an arbitrary function odfx. We deal with gravity only, which do not require the gauge field. We only evaluate particles with no electric charge, which for a neutral particle we obtain the following equations.

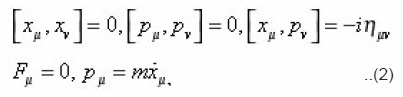
vol9_no1_cha_dha_eq2

Φ(x) = 0 to find the correct geodesic equation.M. Montesinos obtained that the generalization from flat to curved space by taking Eq.(1) as valid in a local Lorenz frame of reference and effect of gravity is brought in by replacing the Minkowskian metric n_μνwith an arbitrary metric g_μν(X). He has shown that this assumption leads to geodesic equation. We choose

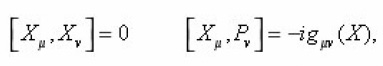
vol9_no1_cha_dha_eq3

Let us assume that the ‘metric’ g_μ(x) is a function of operator X which is a symmetric tensor.

Theorem (Generalization of Commutative Space Time )

Let Xμ(τ) be a new position operator and p_μ(τ) is the corresponding conjugate momenta, mX_μ= P_μ, then solution of equation may be obtain in terms of the operators given in (2). Proof

We construct operators X and P expressed as follows.

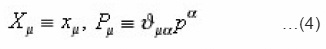
vol9_no1_cha_dha_eq4

Where x_μ and p_ν satisfy relation. By taking the derivative with respect to of Eqs.(4), we obtain the following relation.

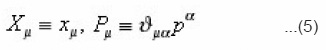
vol9_no1_cha_dha_eq5

Where p_μ = mX_μ . Combining equations (5) and (2) we obtain

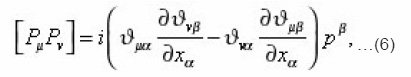
vol9_no1_cha_dha_eq6

vol9_no1_cha_dha_eqA

Combining equations (3), (5), (6), we obtain the equation

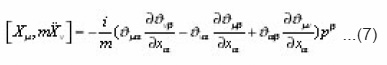
vol9_no1_cha_dha_eq7

By choosing the operator X such that is X_μ = X_μ(x,p), LHS of (7) reduces to the equation

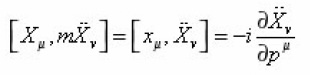
vol9_no1_cha_dha_eqb

We now integrate Eq. (6) over p^μ which gives the relation

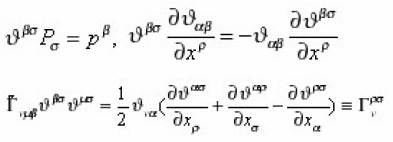
vol9_no1_cha_dha_eqc

By taking G_ν(x) = 0 we get

vol9_no1_cha_dha_eq8

Where all Jacobi identities are satisfied. Eq. (6) is similar to the Christoffel symbol in general relativity, and Eq. (8) is similar to the geodesic equation. Let us assume that ‘metric’ is invertible and define an inverse of the symmetric tensor by the following relation

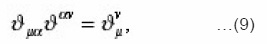
Combining the equations (4), (7) and (9) we get

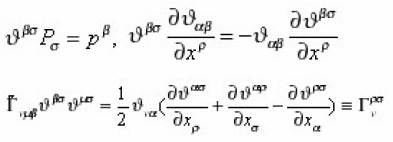
vol9_no1_cha_dha_eqc

Where

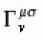
is really the Christoffelsymbol, we get the geodesic equation

vol9_no1_cha_dha_eq10

Where vg_μ tensor is treated only as a symmetric tensor with an inverse defined in Eq. (10).

Theorem (Derivation of Geodric Equation In K- Minkowski Space Time)

We derive the geodesic equation for a particle moving in the non-commutative curved space time and analyze the k-Minkowski deformations of gravity. The methods taken here use k-Minkowskideformations on the flat space time. It is further generalized to k-deformed space time with arbitrary metric.

Proof

Let us define k-Minkowski space by the relation

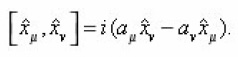
vol9_no1_cha_dha_eqdd

Operators

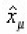
care expressed in terms of operators x and using the derivations due to J. Lukerki and H. Ruegg. We get

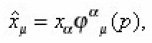
Where

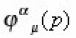
satisfy the identity

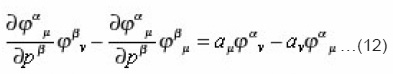
vol9_no1_cha_dha_eq12

Let us solve Eq. (11) up to the first order in deformation parameter a, which gives

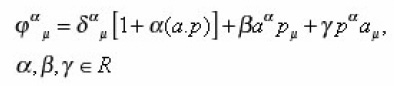
vol9_no1_cha_dha_eqg

Where parameters of the realization a, b, and g satisfy the a constraint

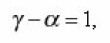
Let us define an operator

which commutes with

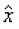
, i.e.

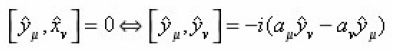
vol9_no1_cha_dha_eqk

Any function of ˆy also commutes with

Where we take only first order in the modified form of give

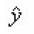
and

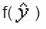
up to the first order in

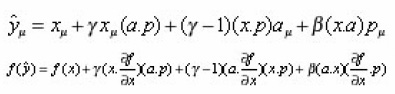
vol9_no1_cha_dha_eqn

The canonical momentum operator ( in e = 0 case )

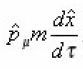
vol9_no1_cha_dha_eqo

as derived by the relation

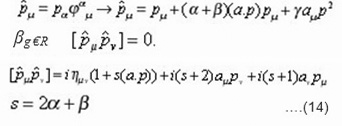
vol9_no1_cha_dha_eq14

Hence , we conclude that construction due to E. HariKumar via Feynman approach satisfy all Jacobi identities. The condition that comes by taking the derivative of Eq. (12) with respect to τ is

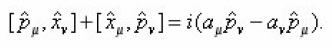
Hence the geodesic equation in the k- Minkowski space time is established.

Theorem ( k-Dependent Corrections to the Geodesic Equation )

Proof

Let us choose the conjugate pairs (x , p ) and for flat non-commutative space time,

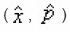
in commutative and non- commutative space respectively. It is shown that all the operators in the flat non-commutative space time are expressed in terms of x , p and deformation parameter a. For the non-commutative space time with curvature. Let us construct it as functions of x , p and deformation parameter . In case of neutral particles , conjugate momenta is given by

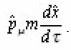
vol9_no1_cha_dha_eqt

We derive the corrections to the geodesic equation due to the k- deformation of space time. Let us consider the relation.

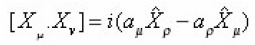
Where

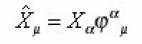
And

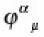
satisfies Eq. (12).

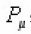
satisfy all the Jacobi Indentifies and the relation

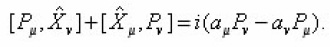
But in the limit a ®0, it implies that

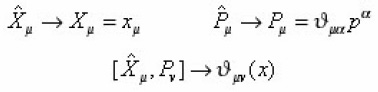
vol9_no1_cha_dha_eqz

By taking limit

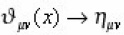
we get the following relations

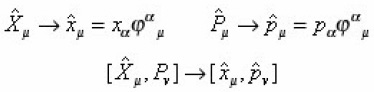
vol9_no1_cha_dha_eq_B

Combining these equation, and comparing it with (13) & (14)

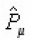
substitute

with a function that commutes with

That is with

Thus , we obtain the required form

By an appropriate application of (14), we find that this construction satisfioes all Jacobi identities and Eq. (15) is also satisfied to all orders in a

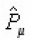
Hence , get its modified form as follows

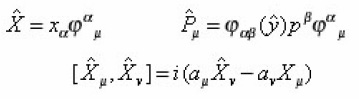
An alternative construction of the operator up to the first order in the deformation parameter may be obtained by differentiating Eq. (17) with respect to τ.

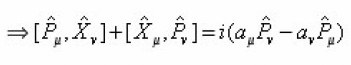
vol9_no1_cha_dha_eq_H

It satisfies antisymmetric part of

We write it as follows

Where

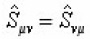
and

Combining Eq. (16) and (17), We get

By taking the limit a a →0, we obtain

Hence , the case of up to the first order in the deformation parameter a

i.e.

Here

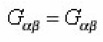
and we get the following relations

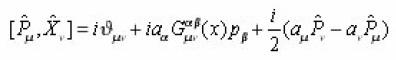
vol9_no1_cha_dha_eq_Q

We get constraints on

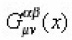
that the Jacobi identities must be satisfied up to the first order in a.

We get

Let us construct

from

and

vol9_no1_cha_dha_eq_W

and express it as

vol9_no1_cha_dha_eq_X

Hence

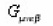
is determined by the parameters a and b and four

More free parameters. This general used form but valid up to the first order in the deformation

parameter a. The construction

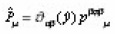
where is the special case of this general procedure.

Conclusion

The principal characteristic of this approach is that all the corrections depend on the choice of realization of the parameters on the mass of the test particle.

We analysed here the a-dependent correction to the Newtonian limit of the geodesic equation which shows that the Newtonian force/potential remains radial, but depends on the mass of the test particle.

We have shown here that the κ-deformed commutation relations between phase space variables induce modified incertainty relations

It has been shown that the commutative limit

gives rise to the metric

and by analogy, we interpret

non- commutative metric .

References

H.S Synder, Phys. Rev. 7: 1-38 (1947).
R. Wald, General relativity University of Chicago Press (1948).
Jacques Bros., The Geometry of Relativistic Space time Seminaire Poincare1 (1-45) (2005).
S. Caroll, Space time and Geometry an Introduction to general relativity (2003).
J. Lukierski and H. Ruegg. A. Nowieki Phys. Lett. B293: 344 (1992)
CrossRef
S. Kresic-Jurie, S. Meljanae and M. Stojic J. Phys. A42: 365204 (2009)
CrossRef
S. Tanimura, Annals of Physics 220: 229 (1992).
CrossRef
E. HariKumar, M., Mod Sivakumar and N. Srinivas . Phys. Lett. A26: 1103 (2011)